6.6

Consider the representation of the ternary relationship of Figure 6.29a using the binary relationships illustrated in Figure 6.29b (attributes not shown).

Show a simple instance of $E$ , $A$ , $B$ , $C$ , $R_{A}$ , $R_{B}$ , and $R_{C}$ that cannot correspond to any instance of $A$ , $B$ , $C$ , and $R$ .

Modify the E-R diagram of Figure 6.29b to introduce constraints that will guarantee that any instance of $E$ , $A$ , $B$ , $C$ , $R_{A}$ , $R_{B}$ , and $R_{C}$ that satisfies the constraints will correspond to an instance of $A$ , $B$ , $C$ , and $R$ .

Modify the preceding translation to handle total participation constraints on the ternary relationship.

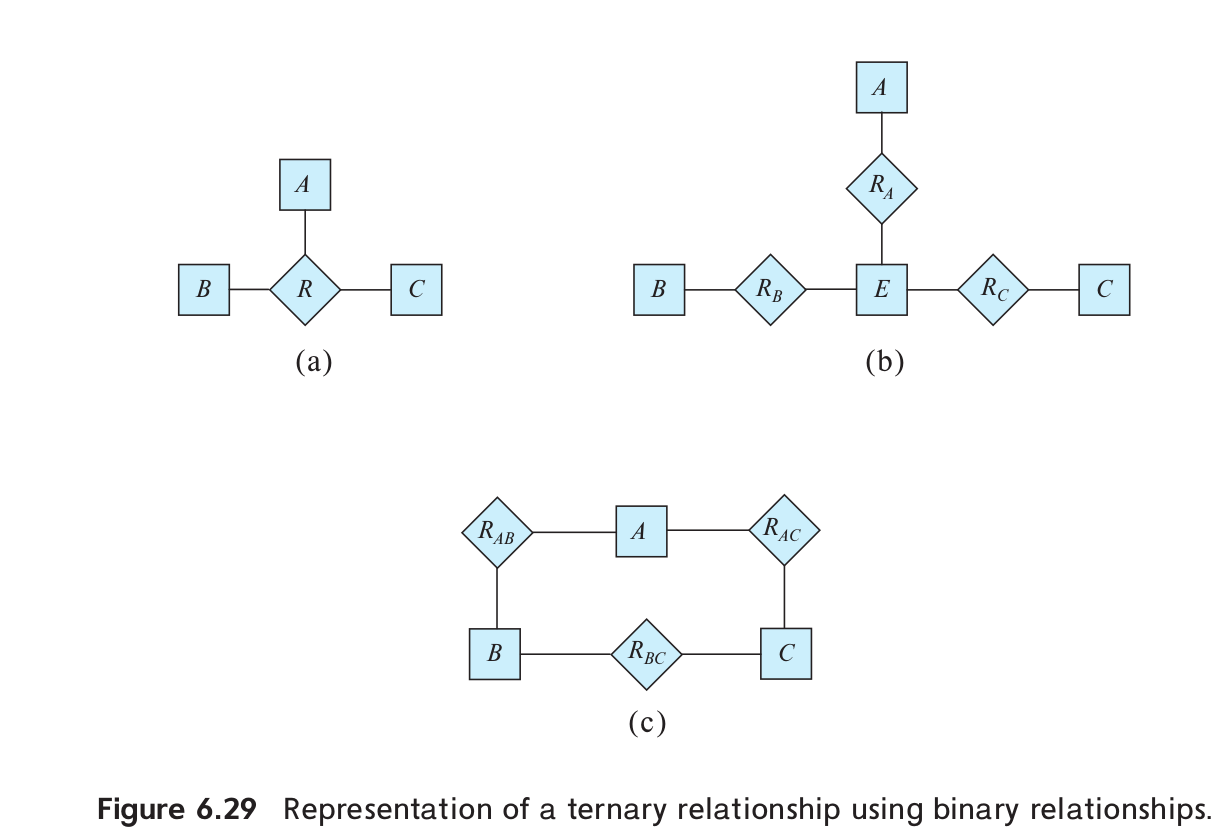
Let $E = {e_{1}, e_{2}}$ , $A = {a_{1}, a_{2}}$ , $B = {b_{1}}$ , $C = {c_{1}}$ , $R_{A} = {(e_{1}, a_{1}), (e_{2}, a_{2})}$ , $R_{B} = {(e_{1}, b_{1})}$ , $R_{C} = {(e_{1}, c_{1})}$ . We see that because of the tuple $(e_{2}, a_{2})$ , no instance of $A$ , $B$ , $C$ , and $R$ exists that correspond to $E$ , $R_{A}$ , $R_{B}$ , and $R_{C}$
See Figure 6.105. The idea is to introduce total participation constraints between $E$ and the relationships $R_{A}$ , $R_{B}$ , $R_{C}$ so that every tuple in $E$ has a relationship with $A$ , $B$ , and $C$ .

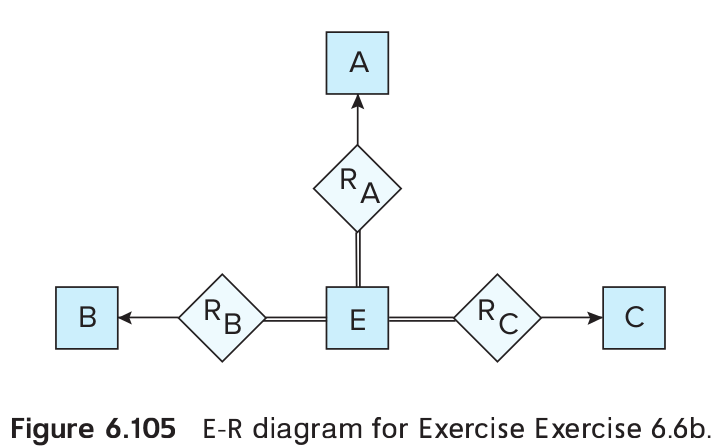
Suppose $A$ totally participates in the relationship $R$ , then introduce a total participation constraint between $A$ and $R_{A}$ , and similarly for $B$ and $C$ .